Domotique

Les Technologies Web

Systèmes Asservis Numériques (Digital Control Systems)

Today, the majority of control systems rely on computers and digital equipment involving controllers. Examples include air/automobile traffic control, chemical and metalworking processes. Thus, conventional control systems are quickly reaching their limits in solving industrial problems or in being well adapted to the modern digital world. In more detail, computers and digital equipment can only understand digital data. This means that computers and digital equipment only take data at fixed intervals. Therefore, it is necessary to develop a method for converting continuous data (time signal) into digital data that can be understood by equipment and computers. 

A digital control system (DCS) can be represented by a block diagram as follows:

Compared to continuous control systems, digital control systems perform very well in the presence of noise, disturbances and interference.

Objectives:

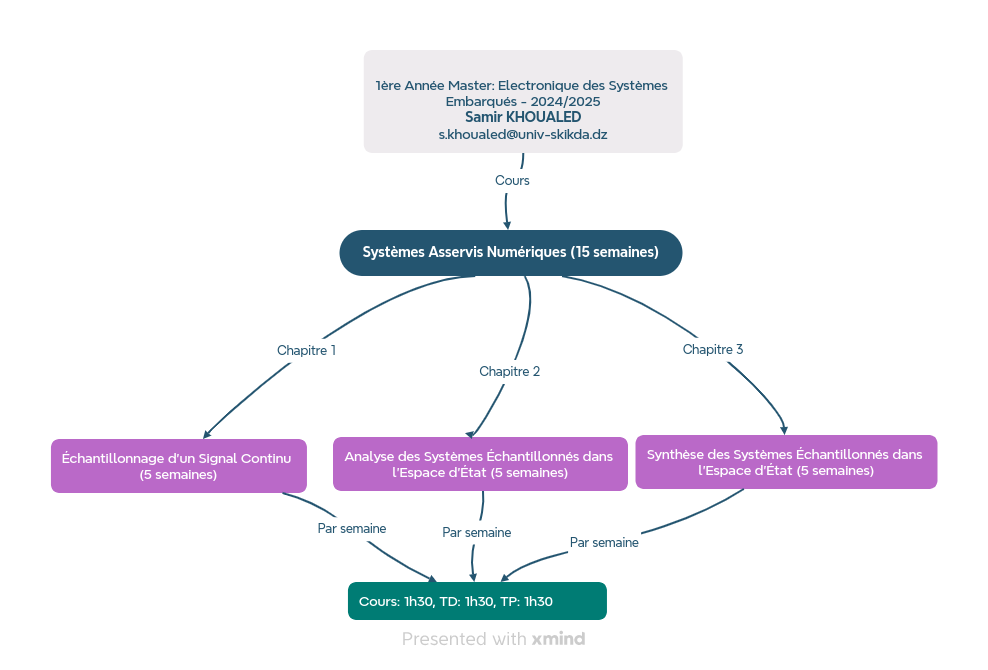
The main objectives of this course are: 

• Understand the advantages of digital control systems (DCS) in industries compared

to old continuous (or analog) systems.

• Understand how to analyze, design and use a DCS.

• Present the properties and representations of linear dynamic systems in discrete

time.

• Give the fundamental elements of the control of linear systems represented in the form

of a Z transfer function.

• Present the different methods of synthesizing discrete time correctors.